This discussion is now closed.
Check out other Related discussions
- A level maths binomial expansion question HELP
- Binomial Expansion Question - A Level Maths
- Binomial Expansion
- weird cosine question
- Maths question
- Binomial expansion- Constant term(Question 2
- Binomial expansion with negative power
- Further Statistics Edexcel 9FM/3B 16th June
- A level Maths question
- Maths statistics question
- Ocr mei unofficial markscheme a level (paper 2 2023)
- Binomial expansion with surds
- OCR A Level Mathematics MEI Pure maths & comprehension H640/03 - 21 Jun 2022 [Chat]
- OCR A-level Mathematics A Paper 1 (H240/01) - 6th June 2023 [Exam Chat]
- Binomial question
- Maclaurin Series - Potential due to Dipole
- Discrete probability disturbution
- Binomial distribution
- Binomial hypothesis test question
- Probability Question
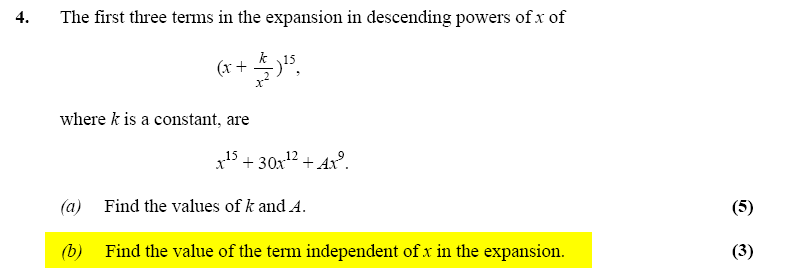
that is, the fomrula for a binomial expansion, putting n=15
So the general term is
Can you find a value of r such that the power of x is zero? (Get the x indices together and solve the equation, or just do it by inspection). Evaluate the general term for this value of r.
nCr. Number of ways of choosing r objects from n items.
Related discussions
- A level maths binomial expansion question HELP
- Binomial Expansion Question - A Level Maths
- Binomial Expansion
- weird cosine question
- Maths question
- Binomial expansion- Constant term(Question 2
- Binomial expansion with negative power
- Further Statistics Edexcel 9FM/3B 16th June
- A level Maths question
- Maths statistics question
- Ocr mei unofficial markscheme a level (paper 2 2023)
- Binomial expansion with surds
- OCR A Level Mathematics MEI Pure maths & comprehension H640/03 - 21 Jun 2022 [Chat]
- OCR A-level Mathematics A Paper 1 (H240/01) - 6th June 2023 [Exam Chat]
- Binomial question
- Maclaurin Series - Potential due to Dipole
- Discrete probability disturbution
- Binomial distribution
- Binomial hypothesis test question
- Probability Question
Latest
Trending
Last reply 1 day ago
Did Cambridge maths students find maths and further maths a level very easy?Last reply 2 weeks ago
Edexcel A Level Mathematics Paper 2 unofficial mark scheme correct me if wrongMaths
71
Trending
Last reply 1 day ago
Did Cambridge maths students find maths and further maths a level very easy?Last reply 2 weeks ago
Edexcel A Level Mathematics Paper 2 unofficial mark scheme correct me if wrongMaths
71